Operational Amplifiers or Op-Amps are considered the workhorse of Analog Electronic Designs. Back from the Analog computers era, Op-Amps have been used for mathematical operations with analog voltages, hence the name operational amplifier. To date, Op-Amps are extensively used for voltage comparison, differentiation, integration, summation and many other things. Needless to say, the Operational Amplifier circuits are very easy to implement for different purposes, but it has a few limitations that often lead to complexity.
The major challenge is to improve the stability of an op-amp in a wide bandwidth of applications. The solution is to compensate the amplifier in terms of frequency response by using a frequency compensation circuit across the operational amplifier. The stability of an amplifier is highly dependent on different parameters. In this article, let’s understand the importance of Frequency Compensation and how to use it in your designs. However, frequency compensation in op amp circuits is crucial for maintaining stability across wide bandwidth applications.
Table of Contents
- What is Frequency Compensation in an Op Amp?
- └ Main Benefits
- Why do we need Frequency Compensation in Op-Amps?
- Open-Loop vs Closed-Loop Configuration
- Types of Op-Amp Frequency Compensation
- External Frequency Compensation in Op Amp
- Types of Op-Amp Compensation Techniques
- Practical simulation
- Troubleshooting Op-Amp Stability Issues
What is Frequency Compensation in an Op Amp?
Before going straight into the advanced application of operational amplifiers and how to stabilise the amplifier using the frequency compensation technique, let's explore a few basic things about the operational amplifier.
An amplifier can be configured as an open-loop configuration or a closed-loop configuration. In an open-loop configuration, there are no feedback circuits associated with it. But in a closed-loop configuration, the amplifier needs feedback to work properly. The operational can have negative feedback or positive feedback. If the feedback network analog across the op-amp’s positive terminal, it is called positive feedback. Otherwise, negative feedback amplifiers have the feedback circuitry connected across the negative terminal. Frequency compensation in an op amp is a technique used to improve amplifier stability by modifying the frequency response characteristics.
Main Benefits of Op-Amp Frequency Compensation
∗ Improved stability under different loading conditions
∗ Improved phase margins (generally >45°)
∗ Reduced ringing effects in switching applications
∗ Improved step response characteristics
∗ Elimination of oscillations caused by capacitive loads
Why do we need Frequency Compensation in Op-Amps?
Let's see the amplifier circuit below. It is a simple negative feedback non-inverting Op-Amp circuit. The circuit is connected as a unity-gain follower configuration.

The above circuit is very common in electronics. As we all know, amplifiers have very high input impedance across the input and could provide a reasonable amount of current across the output. Therefore, operational amplifiers can be driven using low signals to drive loads of higher current.
But what is the maximum current the op-amp could deliver to drive the load safely? The above circuit is good enough to drive pure resistive loads (ideal resistive load), but if we connect a capacitive load across the output, the op-amp will become unstable, and based on the value of load capacitance, in the worst case, the op-amp might even start to oscillate.
Let’s explore why the op-amp gets unstable when a capacitive load is connected across the output. The above circuit can be described as a simple formula -
Acl= A / 1+Aß
Acl is the closed-loop gain. A is the open-loop gain of the amplifier. The β is the feedback factor. That means how much the output is going back to the input of the amplifier. As the amplifier is a unity gain amplifier, the feedback factor is 1; hence, all of the output can be considered as going back to the input.
For the explanation of the Aβ, let's draw the negative feedback amplifier from a different point of view.

The above image is a representation of the formula and negative feedback amplifier circuit. It is identical to the traditional negative amplifier stated previously. They both share AC input on the positive terminal, and both have the same feedback in the negative terminal. The circle is the summing junction that has two inputs, one from the input signal and the second one from the feedback circuit. Well, when the amplifier is working in negative feedback mode, the complete output voltage of the amplifier is flowing through the feedback line to the summing junction point. At the summing junction, the feedback voltage and the input voltage are added together and fed back into the input of the amplifier.
The image is divided into two gain stages. Firstly, it is showing a complete closed-loop circuit, as this is a closed-loop network and also the op-amp's open-loop circuit. Because the op-amp showing A is a standalone open circuit, the feedback is not directly connected.
The output of the summing junction is further amplified by the op-amp open-loop gain. Therefore, if this complete thing is represented as a mathematical formation, the output across the summing junction is -
Vin - Voutβ Now the output of the amplifier will be - Vout = open loop gain x (Vin - Voutβ) Or, Vout = A(Vin - Voutβ) Vout = AVin - VoutAβ Vout + VoutAβ= AVin Vout (1+Aβ) = AVin Vout/Vin = A/(1+Aβ)
Open-Loop vs Closed-Loop Configuration
| Configuration Type | Feedback | Stability | Application |
| Open-Loop | None | High gain, unstable | Limited practical use |
| Closed-Loop Negative | Output to inverting input | Stable with compensation | Most applications |
| Closed-Loop Positive | Output to non-inverting input | Unstable | Oscillators, comparators |
Instability Problem in Op-Amp
Therefore, the above circuit, which is represented as the negative feedback amplifier, has a transfer function of A/1+Aβ, where Aß is the loop gain factor. If in any situation, the 1+Aβ becomes 0, the amplifier's closed-loop gain or the transfer function will become infinity. But the closed-loop gain is limited as the power supply, which is connected across the op-amp, is limited; hence, the Amplifier will become unstable.
Now, for a negative feedback amplifier, the phase shift of the input and output is 180 degrees. When a capacitive load is connected across the amplifier, it can alter the phase by adding a pole across the op-amp output, resulting in a negative-to-positive feedback conversion. The loop gain gets 1 at the 180-degree phase shift and induces instability.
Instability of the amplifier provides poor phase margin and hampered slew rate, which results in an unnatural behaviour across the op-amp output. The output oscillates and creates ringing effects when switching the output state. Since practically there are no ideal loads, resistive loads are not ideally resistive, even perfectly made circuits have lots of capacitance as well as inductance. The outcome is poor phase response at high frequency and instability.
How to deal with Op-Amp Instability?
The solution is not straightforward way. To find out the problem or to check whether the amplifier is stable or not, one needs to find out the amplifier’s phase at unity loop gain. The Solution is to provide frequency compensation to the op-amp. This is a useful technique to overcome the instability of the op-amp as well as improve the step response of the circuit.
Types of Op-Amp Frequency Compensation
There are different types of frequency compensation techniques used in electronics. However, all techniques are categorised into two basic types of compensation techniques. The first one is external compensation across the op-amp, and the second one is the internal compensation technique.
External Frequency Compensation in Op Amp
External compensation techniques vary depending on the application, type of amplifier used and many other things. The easiest way is to use the out-of-loop compensation technique or the in-loop compensation technique.
The out-of-the-loop compensation technique uses a simple resistor to isolate the capacitive load from the op-amp, lowering the capacitive loading of the op-amp. The resistor typically varies from 10 to 50 Ohms, but the increase in the isolated resistor affects the op-amp bandwidth. The bandwidth of the op-amp drastically reduced to a very low value. One of the popular ways of out-of-the-loop frequency compensation techniques is to use the Dominant pole compensation technique.
1. Dominant pole Compensation
This technique uses a simple RC network connected across the output of the operational amplifier circuit. A sample dominant pole compensation circuit is shown below.

This works great to overcome the instability issue. The RC network creates a pole at unity or 0dB gain that dominates or cancels out other high-frequency poles. The transfer function of the dominant pole configuration is –

Where A(s)is the uncompensated transfer function, A is the open-loop gain, and ώ1,ώ2, and ώ3 are the frequencies where the gain roll-off is at -20dB, -40dB, and -60dB, respectively. The Bode plot below shows what happens if the dominant pole compensation technique is added across the op-amp output, where fd is the dominant pole frequency.

2. Miller compensation
Another effective compensation technique is the Miller compensation technique, and it is an in-loop compensation technique where a simple capacitor is used with or without a load isolation resistor (Nulling resistor). That means a capacitor is connected in the feedback loop to compensate for the op-amp frequency response.
The miller compensation circuit is shown below. In this technique, a capacitor is connected to the feedback with a resistor across the output.

The circuit is a simple negative feedback amplifier with inverting gain dependent on R1 and R2. The R3 is the null resistor, and the CL is the capacitive load across the op-amp output. CF is the feedback capacitor, which is used for compensation purposes. The Capacitor and the resistor value depend on the type of amplifier stages, pole compensation, and the capacitive load.
Types of Op-Amp Compensation Techniques
Modern operational amplifiers have an internal compensation technique. In the internal compensation technique, a small feedback capacitor is connected inside the op-amp IC between the second stage's Common emitter transistor. For example, the image below is the internal diagram of the popular op-amp LM358. Modern op-amps like LM358 and LM741 incorporate internal frequency compensation.

The Cc capacitor is connected across the Q5 and Q10. It is the compensation Capacitor (Cc). This compensation capacitor improves the stability of the amplifier and also prevents the oscillation and ringing effect across the output.
Frequency Compensation of Op-amp – Practical simulation
Dominant pole compensation op-amp uses an RC network at the output to create a dominant low-frequency pole that overrides higher-frequency poles. To understand Frequency compensation more practically, let’s try to simulate it by considering the circuit below –

The circuit is a simple negative feedback amplifier using LM393. This op-amp does not have a compensation capacitor built in. We will simulate the circuit in Pspice with a 100pF capacitive load and will check how it will perform in low and high-frequency operation.
To check this, one needs to analyse the open-loop gain and phase margin of the circuit. But it is a bit tricky for the PSPICE since simulating the exact circuit, as shown above, will represent its closed-loop gain. Therefore, special considerations need to be taken. The step to converting the above circuit for open-loop gain simulation (gain vs phase) in PSPICE is stated below,
- The input is grounded to obtain the feedback response; closed-loop input to output is ignored.
- Inverting input is broken into two parts. One is the voltage divider, and another one is the negative terminal of the op-amp.
- Two parts are renamed to create two separate nodes for identification purposes during the simulation phase. The voltage divider section is renamed as feedback, and the negative terminal is renamed as Inv-input. (Inverting input).
- These two broken nodes are connected with a 0V DC voltage source. This is done because, in terms of DC voltage, both nodes have the same voltage, which is essential for the circuit to satisfy the current operating point requirement.
- Adding the voltage source with a 1V AC stimulus. This forces the two individual nodes' voltage difference to become 1 during the AC analysis. One thing is essential in this case: that the ratio of the feedback and the inverting input is dependent on the circuit's open-loop gain.
After making the above steps, the circuit looks like this -

The circuit is powered using a 15V +/- power supply rail. Let's simulate the circuit and check its output Bode plot.

Since the circuit has no frequency compensation, as expected, the simulation is showing high gain at low frequency and low gain at high frequency. Also, it is showing a very poor phase margin. Let's see what the phase is at 0dB gain.

As you can see, even at 0dB gain or unity gain crossover, the op-amp is providing 6 degrees of phase shift at just 100pF capacitive load.
Now let’s improvise the circuit by adding a frequency compensation resistor and capacitor to create Miller compensation across the op-amp and analyse the result. A 50 Ohms of null resistor is placed across the op-amp and the output with a 100pF compensation capacitor. Miller compensation places a compensation capacitor op amp in the feedback path, utilising the Miller effect to enhance stability.

The simulation is done, and the curve looks like the one below,

The Phase curve is much better now. The phase shift at 0dB gain is almost 45.5 degrees. The amplifier stability is highly increased using the frequency compensation technique. Therefore, it is proven that the frequency compensation technique is highly recommended for the better stability of the op-map. But the Bandwidth will decrease.

Now we understand the importance of frequency compensation of the op-amp and how to use it in our Op-Amp designs to avoid instability problems. Hope you enjoyed reading the tutorial and learnt something useful. If you have any questions, leave them in our forums or in the comment section below.
Troubleshooting Op-Amp Stability Issues
| Problem | Symptom | Solution |
| Oscillation | High-frequency noise | Add compensation capacitor |
| Ringing | Overshoot in step response | Increase damping resistance |
| Poor bandwidth | Slow response | Optimize compensation values |
| Phase shift | Instability with load changes | Use Miller compensation |
Conclusion
Frequency compensation of op amp circuits is a necessary aspect of any reliable analog circuit design. Whether you are using internal frequency compensation, as typically found on modern-day op-amps, or whether you are using an external frequency compensation technique such as dominant pole compensation or out-of-phase Miller compensation, any stabilisation will guarantee reliable performance across any operating condition.
Selecting which method of op-amp compensation techniques to use for your application is dependent on application specifications, load characteristics, and performance objectives.
Frequently Asked Questions on Op-amp Compensation Techniques
⇥ 1. What is dominant pole compensation, and how is it accomplished in op-amps?
Dominant pole compensation is formed by establishing a low-frequency pole with RC network components. The frequency response is shaped as it is reduced by -20dB/decade, and gives a phase margin for frequency response counter to instability.
⇥ 2. What is Miller compensation in op-amp circuits?
Miller compensation utilises a capacitor in the feedback path that takes advantage of the Miller effect to produce a dominant pole, thus doubling the compensation capacitor values, thereby improving stability with little external components.
⇥ 3. How do you find out the values of the compensation capacitors?
Values of the compensation capacitors can be calculated with the equation C=1/(2πRf), where R is the isolation resistance and f is the desired dominant pole frequency. For this, we normally select f for the 45° phase margin.
⇥ 4. What is wrong with an op-amp without the correct compensation?
Without compensation, op-amps can oscillate, exhibit ringing in step response, low phase margin (<45°), or become unstable when driving capacitive loads, rendering the circuit unusable.
⇥ 5. Which of these op-amps are internally compensated?
Examples of well-known internally compensated op-amps are LM741, LM358, LF356, and most general-use op-amps. Speciality high-speed, or high-precision op-amps typically require external compensation to achieve the best results.
⇥ 6. What impact does load capacitance have on op-amp stability?
Load capacitance adds an extra pole to the frequency response and creates phase shifts, resulting in instability. Larger capacitance values will require more vigorous compensation to maintain stability margins.
Explore Op-amp Based Projects
You can find various projects where we applied an Op-amp. Browse the links below to discover them.
How to Design and Build a Simple Monostable Multivibrator Circuit using an Op-amp
In this tutorial, we will be making an Op-amp-based monostable multivibrator circuit along with all its calculations and testing. So let’s get right into it.
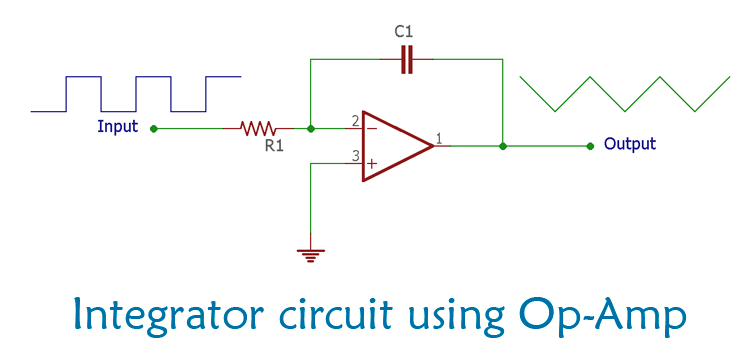
Operational Amplifier Integrator Circuit: Construction, Working and Applications
An op-amp or Operational Amplifier is the backbone of Analog Electronics, and out of many applications, such as a Summing Amplifier, a differential amplifier, and an Instrumentation Amplifier, an Op-Amp can also be used as an integrator, which is a very useful circuit in analog-related applications.
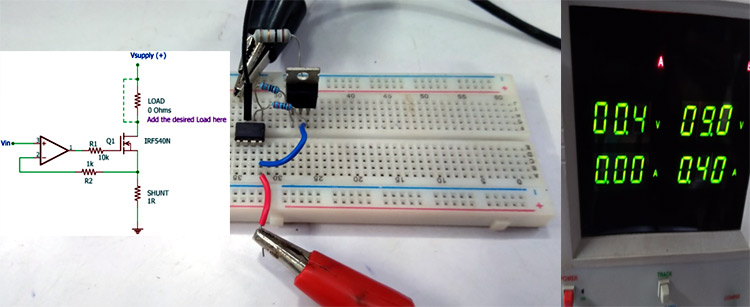
Design a Voltage Controlled Current Source Circuit using an Op-Amp
This comprehensive guide demonstrates how to design and build a voltage-controlled current source using an op amp with practical examples. This type of voltage-controlled current source circuit is also called a current servo.